Abstract
The technique of relaxed power series expansion provides an efficient
way to solve equations of the form  ,
where the unknown
,
where the unknown  is a
vector of power series, and where the solution can be obtained as the
limit of the sequence
is a
vector of power series, and where the solution can be obtained as the
limit of the sequence 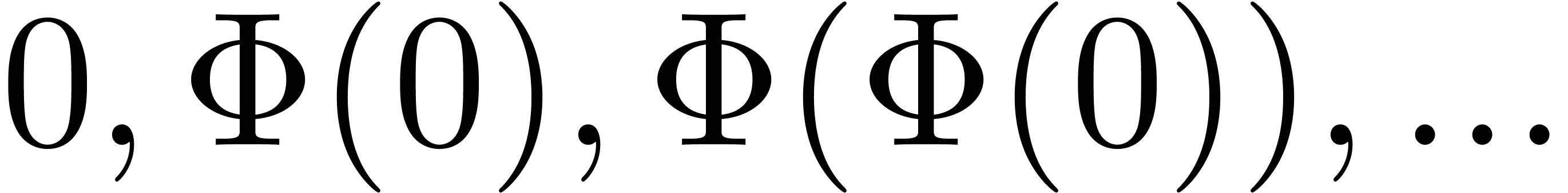 . With
respect to other techniques, such as Newton's method, two major
advantages are its generality and the fact that it takes advantage of
possible sparseness of
. With
respect to other techniques, such as Newton's method, two major
advantages are its generality and the fact that it takes advantage of
possible sparseness of  . In
this paper, we extend the relaxed expansion mechanism to more general
implicit equations of the form
. In
this paper, we extend the relaxed expansion mechanism to more general
implicit equations of the form 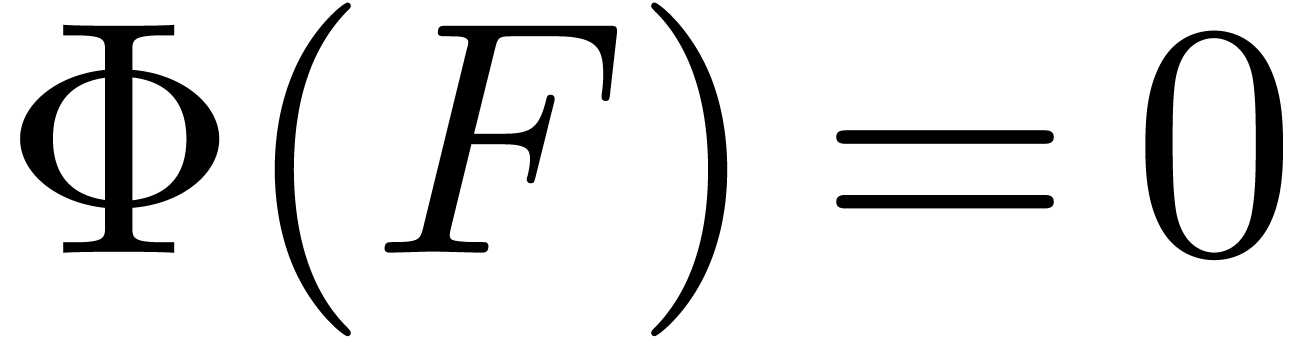 .
.
Occasion: ACA 2010, Vlora, Albania, June 2010
Documents: slideshow, TeXmacs
source
 ,
where the unknown
,
where the unknown  is a
vector of power series, and where the solution can be obtained as the
limit of the sequence
is a
vector of power series, and where the solution can be obtained as the
limit of the sequence 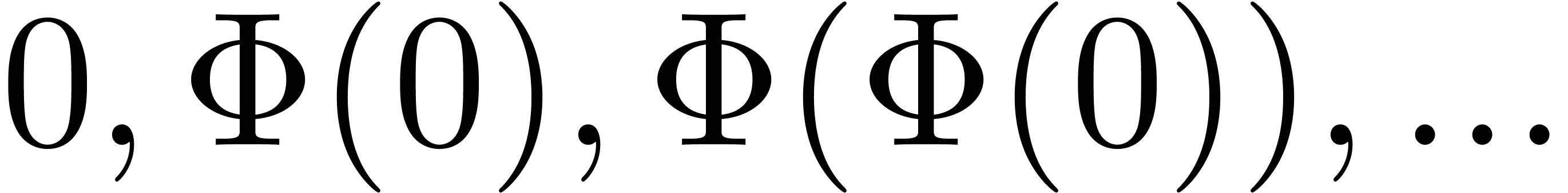 . With
respect to other techniques, such as Newton's method, two major
advantages are its generality and the fact that it takes advantage of
possible sparseness of
. With
respect to other techniques, such as Newton's method, two major
advantages are its generality and the fact that it takes advantage of
possible sparseness of  . In
this paper, we extend the relaxed expansion mechanism to more general
implicit equations of the form
. In
this paper, we extend the relaxed expansion mechanism to more general
implicit equations of the form 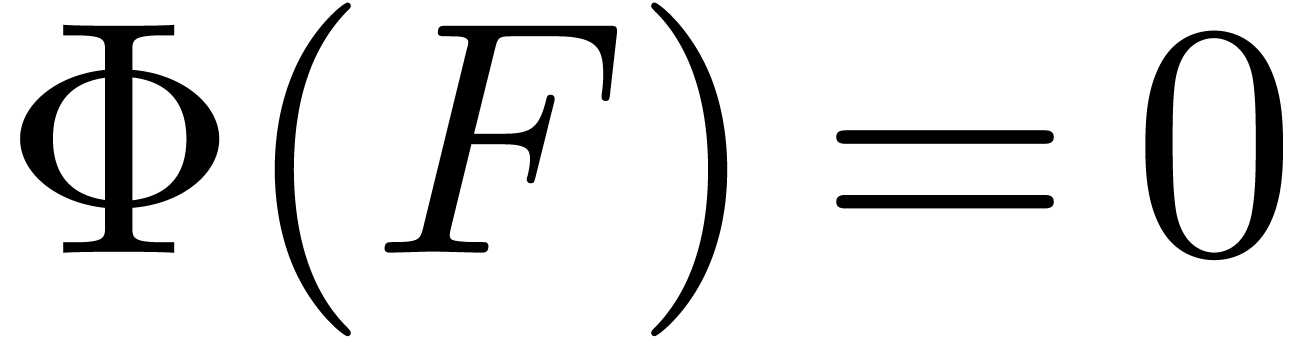 .
.